Formula For Finite Geometric Series Math
Pin On Math Easy Tutorials
Boolean Logic Basic Lexicon Of Translational Equivalents Algebra Partial Differential Equation
Interactive Notebook Arithmetic And Geometric Sequence Series Math Notebooks Notes Concepts
Geometric Series Examples Solutions Videos Worksheets Games Activities
Https Www Kentschools Net Wp Content Blogs Dir 130 Files 2017 04 8 3 New Pdf
Lesson Geometric Sequences Nagwa
Derivation Of The Formula For Sum A Geometric Series Youtube
Pin On Printable Blank Worksheet Template
The first term of an geometric progression is 1 and the common ratio is 5 determine how many terms must be added together to give a sum of 3906.
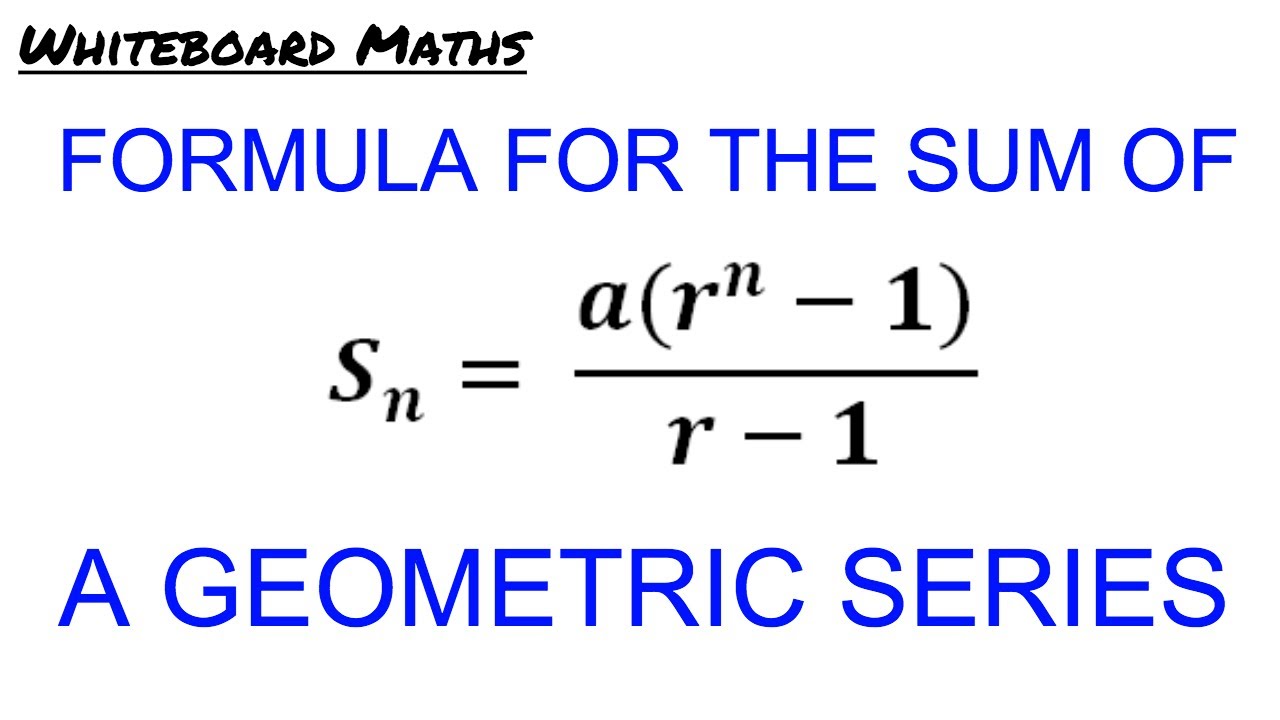
Formula for finite geometric series math. You will get the value of a with this method. In this video sal gives a pretty neat justification as to why the formula works. The nth term from the end of the gp with the last term l and common ratio r l r n 1. Geometric series are among the simplest examples of infinite series with finite sums although not all of them have this property.
Swing our mission is to provide a free world class education to anyone anywhere. Khan academy is a 501 c 3 nonprofit organization. If you re seeing this message it means we re having trouble loading external resources on our website. The general formula for determining the sum of a geometric series is given by.
In the case of the geometric series you just need to specify the first term a and the constant ratio r. Find the common ratio if the fourth term in geometric series is frac 4 3 and the eighth term is frac 64 243. In mathematics a geometric series is a series with a constant ratio between successive terms for example the series is geometric because each successive term can be obtained by multiplying the previous term by 1 2. A 10 the first term r 3 the common ratio n 4 we want to sum the first 4 terms.
Sn a 1 r n 1 r where r 1 this formula is easier to use when r 1. Plugging into the geometric series sum formula i get. S 4 a 1 r 4 1 r mathrm s 4 a left dfrac 1 r 4 1 r right s4. In general in order to specify an infinite series you need to specify an infinite number of terms.
The values of a r and n are. Sn a rn 1 r 1 if r 1and r 1. Sn a 1 rn 1 r if r 1 and r 1. Geometric series word problems.
S sum till n terms n number of terms r common ratio a first term if you have to find the first term given the sum and common ratio substitute the value of s r and n and do the calculations carefully. The formula to calculate the sum of the first n terms of a gp is given by.
Arithmetic Sequences Hsf Bf A 1 2 Editable 48 Questions Game Activities
Finding The Sum Of A Finite Geometric Series Youtube
Http Cavanaughmath Pbworks Com W File Fetch 65105741 Precalc 20lesson 2012 3 Pdf
Your Algebra 2 Students Will Write Analyze And Evaluate A Finite Geometric Series Solve Real World Situations With Seri
Pin On Math Lessons Notes Powerpoints Assignments
Geometric Series Proof Of The Sum First N Terms Examsolutions Youtube
Arithmetic And Geometric Sequence Sum Nth Term Cheat Sheet Foldable Sequences Math Notebooks
Http Skwon Org Colalgres Mlarcolalg8 08 03 Pdf
Finite And Infinite Geometric Series Youtube
Introduction To Sequences 23 Surefire Examples Geometric Series Math Methods
How To Find Arithmetic And Geometric Series 13 Surefire Examples Sequences Math Methods
Find The Number Of Terms In A Geometric Sequence Youtube